Android游戲開發之黑白棋

黑白棋介紹

黑白棋，又叫蘋果棋，最早流行於西方國家。游戲通過相互翻轉對方的棋子，最後以棋盤上誰的棋子多來判斷勝負。黑白棋非常易於上手，但精通則需要考慮許多因素，比如角邊這樣的特殊位置、穩定度、行動力等。本游戲取名為黑白棋大師，提供了8種難度等級的選擇，從菜鳥、新手、入門、棋手到棋士、大師、宗師、棋聖，助你不斷提升棋力。

黑白棋游戲規則

游戲規則見黑白棋大師中的截圖。

黑白棋大師游戲截圖

游戲啟動界面。

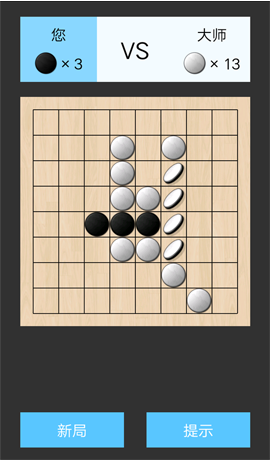
游戲過程中的一個截圖。

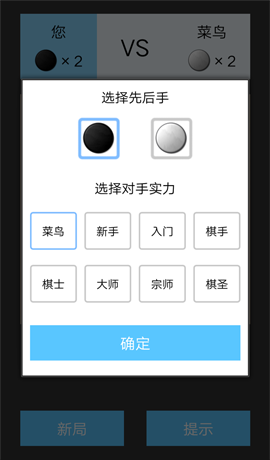
開新局時的選項，選擇先後手以及AI的水平。

幾個關鍵的類

Rule

Rule類實現游戲規則相關的方法，包括

    1.判斷某一步是否合法

    2.獲取所有的合法走步

    3.走一步並翻轉敵方棋子

    4.統計兩方棋子個數

Algorithm

Algorithm類實現極小極大算法，包括

    1.局面評估函數，對當前局面打分，越高對max越有利，越低對min越有利

    2.min()方法

    3.max()方法

    4.獲得一個好的走步

ReversiView

ReversiView繼承自SurfaceView，實現棋盤的界面，在該類定義棋盤界面的繪制、更新等操作。

RenderThread

RenderThread繼承自Thread，是控制ReversiView以一定fps更新、重繪界面的線程。

具體實現

棋盤表示

byte[][]二維數組存儲棋盤，-1表示有黑子，1表示有白子，0表示棋格為空

游戲規則類Rule的實現

提供幾個關於游戲規則的靜態方法。

判斷某一個位置是否位於棋盤內

public static boolean isLegal(int row, int col) {
  return row >= 0 && row < 8 && col >= 0 && col < 8;
}

判斷某一方在某個位置落子是否合法

即判斷該子是否能與己方棋子在某個方向上夾住敵方棋子。

public static boolean isLegalMove(byte[][] chessBoard, Move move, byte chessColor) {
    int i, j, dirx, diry, row = move.row, col = move.col;
    if (!isLegal(row, col) || chessBoard[row][col] != Constant.NULL)
      return false;
    for (dirx = -1; dirx < 2; dirx++) {
      for (diry = -1; diry < 2; diry++) {
        if (dirx == 0 && diry == 0) continue;
        int x = col + dirx, y = row + diry;
        if (isLegal(y, x) && chessBoard[y][x] == (-chessColor)) {
          for (i = row + diry * 2, j = col + dirx * 2; isLegal(i, j); i += diry, j += dirx) {
            if (chessBoard[i][j] == (-chessColor)) {
              continue;
            } else if (chessBoard[i][j] == chessColor) {
              return true;
            } else {
              break;
            }
          }
        }
      }
    }
    return false;
}

某一方走一步子

將各個方向上被翻轉的棋子的顏色改變，並返回這些棋子在棋盤的位置，方便顯示翻轉動畫。

public static List<Move> move(byte[][] chessBoard, Move move, byte chessColor) {
  int row = move.row;
  int col = move.col;
  int i, j, temp, m, n, dirx, diry;
  List<Move> moves = new ArrayList<Move>();
  for (dirx = -1; dirx < 2; dirx++) {
    for (diry = -1; diry < 2; diry++) {
      if (dirx == 0 && diry == 0)
        continue;
      temp = 0;
      int x = col + dirx, y = row + diry;
      if (isLegal(y, x) && chessBoard[y][x] == (-chessColor)) {
        temp++;
        for (i = row + diry * 2, j = col + dirx * 2; isLegal(i, j); i += diry, j += dirx) {
          if (chessBoard[i][j] == (-chessColor)) {
            temp++;
            continue;
          } else if (chessBoard[i][j] == chessColor) {
            for (m = row + diry, n = col + dirx; m <= row + temp && m >= row - temp && n <= col + temp
                && n >= col - temp; m += diry, n += dirx) {
              chessBoard[m][n] = chessColor;
              moves.add(new Move(m, n));
            }
            break;
          } else
            break;
        }
      }
    }
  }
  chessBoard[row][col] = chessColor;
  return moves;
}

獲取某一方當前全部合法的落子位置

public static List<Move> getLegalMoves(byte[][] chessBoard, byte chessColor) {
  List<Move> moves = new ArrayList<Move>();
  Move move = null;
  for (int row = 0; row < 8; row++) {
    for (int col = 0; col < 8; col++) {
      move = new Move(row, col);
      if (Rule.isLegalMove(chessBoard, move, chessColor)) {
        moves.add(move);
      }
    }
  }
  return moves;
}

統計玩家和AI的棋子個數

public static Statistic analyse(byte[][] chessBoard, byte playerColor) {

  int PLAYER = 0;
  int AI = 0;
  for (int i = 0; i < 8; i++) {
    for (int j = 0; j < 8; j++) {
      if (chessBoard[i][j] == playerColor)
        PLAYER += 1;
      else if (chessBoard[i][j] == (byte)-playerColor)
        AI += 1;
    }
  }
  return new Statistic(PLAYER, AI);
}

游戲算法類Algorithm的實現

極大過程和極小過程

這兩個過程的函數形式為：

復制代碼 代碼如下:
private static MinimaxResult max(byte[][] chessBoard, int depth, int alpha, int beta, byte chessColor, int difficulty);
private static MinimaxResult min(byte[][] chessBoard, int depth, int alpha, int beta, byte chessColor, int difficulty);

chessBoard為棋盤；depth為博弈樹搜索深度；alpha和beta用於alpha-beta剪枝，在max方法中alpha不斷更新為局面評分的較大值，在min方法中beta不斷更新為局面評分的較小值，當alpha >= beta時就進行剪枝；chessColor表示棋子顏色；difficulty表示游戲難度，對應於不同的AI水平。

由於黑子先行，黑子總是調用max()方法，白子調用min()方法。

下面以極大過程為例。

如果深度為0，只要返回當前局面評分即可。如果雙方均沒有步可走，表示已經達到最終局面，返回該局面評分。如果僅單方無處可走，調用min遞歸即可。

正常情況下有步可走，遍歷每個合法的走步，如果alpha大於等於beta，剪枝直接break，否則走步並遞歸。

best是當前max節點維護的一個最佳值，調用的min方法的alpha是取得alpha和best的較大值。

private static MinimaxResult max(byte[][] chessBoard, int depth, int alpha, int beta, byte chessColor, int difficulty) {
  if (depth == 0) {
    return new MinimaxResult(evaluate(chessBoard, difficulty), null);
  }
  List<Move> legalMovesMe = Rule.getLegalMoves(chessBoard, chessColor);
  if (legalMovesMe.size() == 0) {
    if (Rule.getLegalMoves(chessBoard, (byte)-chessColor).size() == 0) {
      return new MinimaxResult(evaluate(chessBoard, difficulty), null);
    }
    return min(chessBoard, depth, alpha, beta, (byte)-chessColor, difficulty);
  }
  byte[][] tmp = new byte[8][8];
  Util.copyBinaryArray(chessBoard, tmp);
  int best = Integer.MIN_VALUE;
  Move move = null;

  for (int i = 0; i < legalMovesMe.size(); i++) {
    alpha = Math.max(best, alpha);
    if(alpha >= beta){
      break;
    }
    Rule.move(chessBoard, legalMovesMe.get(i), chessColor);
    int value = min(chessBoard, depth - 1, Math.max(best, alpha), beta, (byte)-chessColor, difficulty).mark;
    if (value > best) {
      best = value;
      move = legalMovesMe.get(i);
    }
    Util.copyBinaryArray(tmp, chessBoard);
  }
  return new MinimaxResult(best, move);
}

private static MinimaxResult min(byte[][] chessBoard, int depth, int alpha, int beta, byte chessColor, int difficulty) {
  if (depth == 0) {
    return new MinimaxResult(evaluate(chessBoard, difficulty), null);
  }
  List<Move> legalMovesMe = Rule.getLegalMoves(chessBoard, chessColor);
  if (legalMovesMe.size() == 0) {
    if (Rule.getLegalMoves(chessBoard, (byte)-chessColor).size() == 0) {
      return new MinimaxResult(evaluate(chessBoard, difficulty), null);
    }
    return max(chessBoard, depth, alpha, beta, (byte)-chessColor, difficulty);
  }
  byte[][] tmp = new byte[8][8];
  Util.copyBinaryArray(chessBoard, tmp);
  int best = Integer.MAX_VALUE;
  Move move = null;

  for (int i = 0; i < legalMovesMe.size(); i++) {
    beta = Math.min(best, beta);
    if(alpha >= beta){
      break;
    }
    Rule.move(chessBoard, legalMovesMe.get(i), chessColor);
    int value = max(chessBoard, depth - 1, alpha, Math.min(best, beta), (byte)-chessColor, difficulty).mark;
    if (value < best) {
      best = value;
      move = legalMovesMe.get(i);
    }
    Util.copyBinaryArray(tmp, chessBoard);
  }
  return new MinimaxResult(best, move);
}

alpha-beta剪枝原理

先解釋下alpha和beta的物理含義，alpha表示max節點迄今為止的最佳局面評分，beta表示min節點迄今為止的最佳局面評分。

舉個例子見下圖（數值為虛構），假設深度是兩層，每個結點有兩行數字，上方的兩個數分別是alpha和beta，表示作為參數傳到該層的alpha和beta。下方的數表示了該節點best的更新過程。

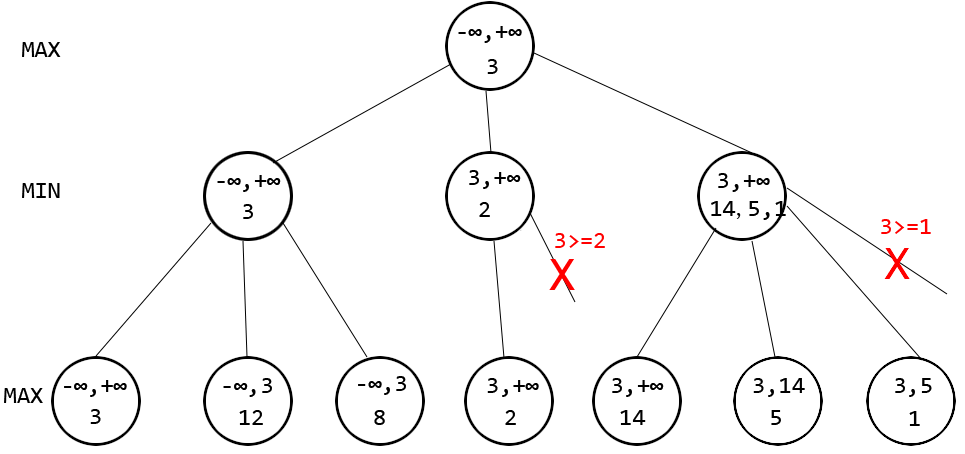
看圖中第一個紅色的叉號，該位置處會更新beta為正無窮和2的較小值，即2，導致alpha大於等於beta成立，發生剪枝，對應於min方法中相應位置處的break操作。

獲得AI計算出的最佳走步

該方法用於AI走步以及提示功能。

public static Move getGoodMove(byte[][] chessBoard, int depth, byte chessColor, int difficulty) {
    if (chessColor == Constant.BLACK)
      return max(chessBoard, depth, Integer.MIN_VALUE, Integer.MAX_VALUE, chessColor, difficulty).move;
    else
      return min(chessBoard, depth, Integer.MIN_VALUE, Integer.MAX_VALUE, chessColor, difficulty).move;
}

局面評估函數

局面評估函數決定了AI水平的高低。對應於不同的AI等級，設計了不同的評估函數。

菜鳥級別只關注棋子個數，新手、入門、棋手3個級別不僅關注棋子的個數，而且關注特殊位置的棋子（邊、角），棋士和大師級別在棋子個數、邊角之外還考慮了行動力，即對方下輪可選的下子位置的個數，宗師和棋聖考慮穩定度和行動力。穩定度將在下一小節介紹。

private static int evaluate(byte[][] chessBoard, int difficulty) {
    int whiteEvaluate = 0;
    int blackEvaluate = 0;
    switch (difficulty) {
    case 1:
      for (int i = 0; i < 8; i++) {
        for (int j = 0; j < 8; j++) {
          if (chessBoard[i][j] == WHITE) {
            whiteEvaluate += 1;
          } else if (chessBoard[i][j] == BLACK) {
            blackEvaluate += 1;
          }
        }
      }
      break;
    case 2:
    case 3:
    case 4:
      for (int i = 0; i < 8; i++) {
        for (int j = 0; j < 8; j++) {
          if ((i == 0 || i == 7) && (j == 0 || j == 7)) {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 5;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 5;
            }
          } else if (i == 0 || i == 7 || j == 0 || j == 7) {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 2;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 2;
            }
          } else {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 1;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 1;
            }
          }
        }
      }
      break;
    case 5:
    case 6:
      for (int i = 0; i < 8; i++) {
        for (int j = 0; j < 8; j++) {
          if ((i == 0 || i == 7) && (j == 0 || j == 7)) {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 5;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 5;
            }
          } else if (i == 0 || i == 7 || j == 0 || j == 7) {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 2;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 2;
            }
          } else {
            if (chessBoard[i][j] == WHITE) {
              whiteEvaluate += 1;
            } else if (chessBoard[i][j] == BLACK) {
              blackEvaluate += 1;
            }
          }
        }
      }
      blackEvaluate = blackEvaluate * 2 + Rule.getLegalMoves(chessBoard, BLACK).size();
      whiteEvaluate = whiteEvaluate * 2 + Rule.getLegalMoves(chessBoard, WHITE).size();
      break;
    case 7:
    case 8:
      /**
       * 穩定度
       */
      for (int i = 0; i < 9; i++) {
        for (int j = 0; j < 9; j++) {
          int weight[] = new int[] { 2, 4, 6, 10, 15 };
          if (chessBoard[i][j] == WHITE) {
            whiteEvaluate += weight[getStabilizationDegree(chessBoard, new Move(i, j))];
          } else if (chessBoard[i][j] == BLACK) {
            blackEvaluate += weight[getStabilizationDegree(chessBoard, new Move(i, j))];
          }
        }
      }
      /**
       * 行動力
       */
      blackEvaluate += Rule.getLegalMoves(chessBoard, BLACK).size();
      whiteEvaluate += Rule.getLegalMoves(chessBoard, WHITE).size();
      break;
    }
    return blackEvaluate - whiteEvaluate;
}

穩定度計算

我們知道，在黑白棋中，棋盤四角的位置一旦占據是不可能再被翻轉的，因此這幾個位置上的子必然是穩定子，而邊上的子只有可能沿邊的方向被翻轉，穩定的程度高於中間的位置上的子。

因此，試圖給每個子定義一個穩定度，描述該子不被翻轉的穩定程度。

一共有四個方向，即左-右，上-下，左上-右下，右上-左下。舉個例子，下面代碼中的 (drow[0][0], dcol[0][0])表示向左移動一個單位的向量，(drow[0][1], dcol[0][1])表示向右移動一個單位的向量。

對於棋盤中某個子的位置，向左找到第一個不是該顏色的位置（可以是出界），再向右找到第一個不是該顏色的位置（可以是出界），如果這兩個位置至少有一個出界，或者兩個均為敵方棋子，穩定度加1。

對於另外三個方向作同樣操作。可以看到，角上的棋子的穩定度必然為4，其他位置則根據具體情況並不恆定不變。

private static int getStabilizationDegree(byte[][] chessBoard, Move move) {
    int chessColor = chessBoard[move.row][move.col];
    int drow[][], dcol[][];
    int row[] = new int[2], col[] = new int[2];
    int degree = 0;

    drow = new int[][] { { 0, 0 }, { -1, 1 }, { -1, 1 }, { 1, -1 } };
    dcol = new int[][] { { -1, 1 }, { 0, 0 }, { -1, 1 }, { -1, 1 } };

    for (int k = 0; k < 4; k++) {
      row[0] = row[1] = move.row;
      col[0] = col[1] = move.col;
      for (int i = 0; i < 2; i++) {
        while (Rule.isLegal(row[i] + drow[k][i], col[i] + dcol[k][i])
            && chessBoard[row[i] + drow[k][i]][col[i] + dcol[k][i]] == chessColor) {
          row[i] += drow[k][i];
          col[i] += dcol[k][i];
        }
      }
      if (!Rule.isLegal(row[0] + drow[k][0], col[0] + dcol[k][0])
          || !Rule.isLegal(row[1] + drow[k][1], col[1] + dcol[k][1])) {
        degree += 1;
      } else if (chessBoard[row[0] + drow[k][0]][col[0] + dcol[k][0]] == (-chessColor)
          && chessBoard[row[1] + drow[k][1]][col[1] + dcol[k][1]] == (-chessColor)) {
        degree += 1;
      }
    }
    return degree;
}

以上就是Android黑白棋游戲實現過程及代碼解析的全部內容，相信本文對大家開發Android黑白棋游戲很有幫助，謝謝大家對本站的支持。